File:SIR model anim.gif
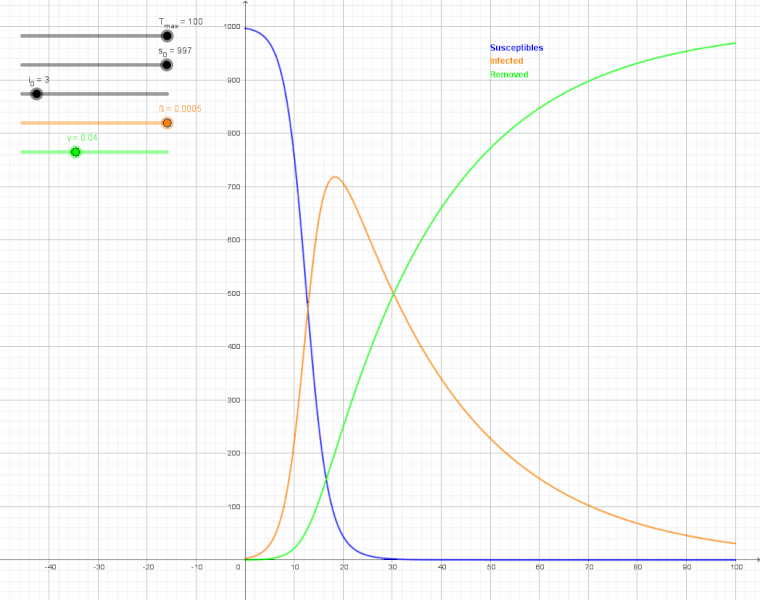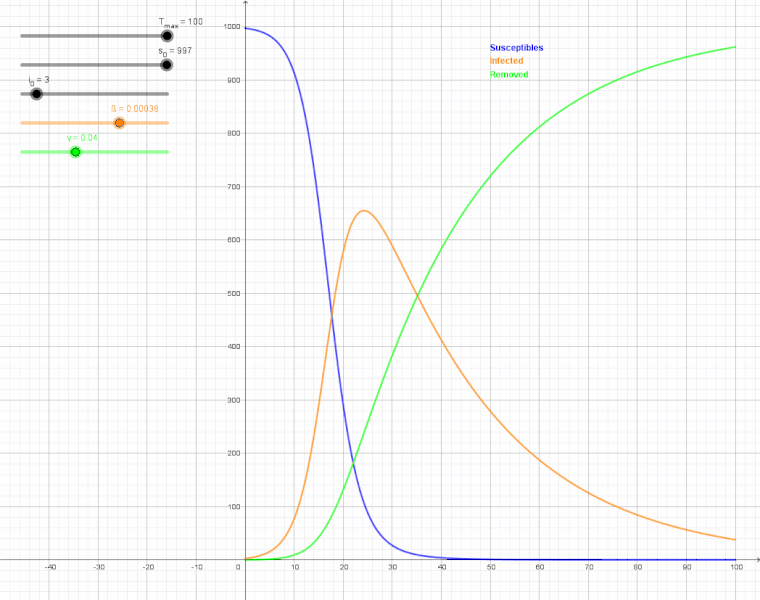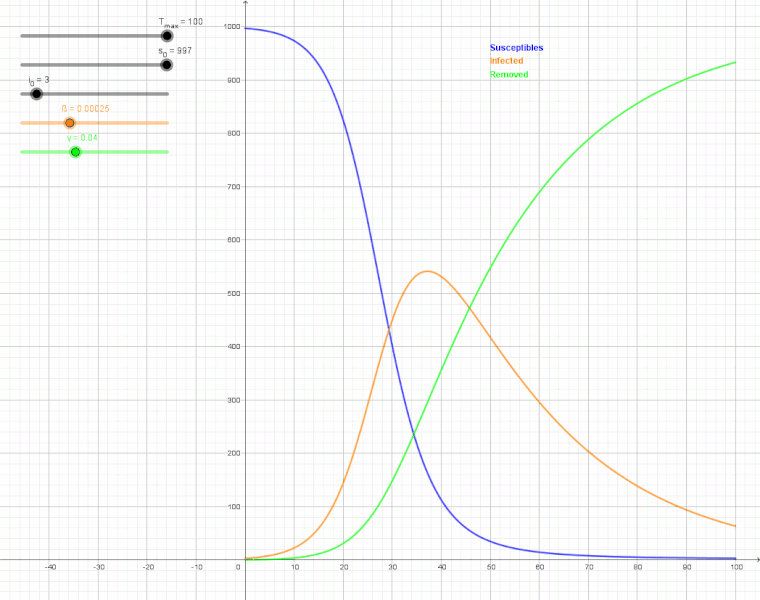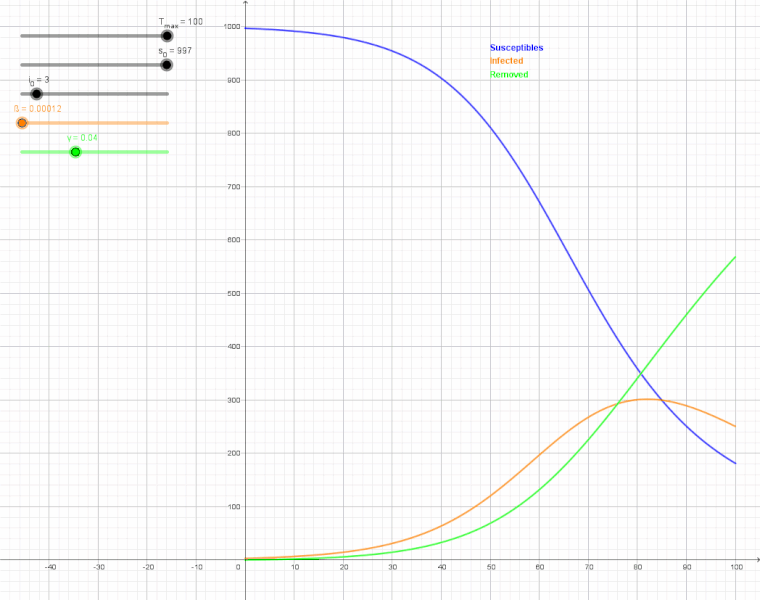
Original file (1,048 × 827 pixels, file size: 4.62 MB, MIME type: image/gif, looped, 77 frames, 0.8 s)
Captions
Captions
Summary
[edit]| DescriptionSIR model anim.gif |
Deutsch: Animation zum SIR-Modell mit den Startwerten
 , ,  , ,  sowie anfänglicher Infektionsrate sowie anfänglicher Infektionsrate  sowie der konstanten Rate sowie der konstanten Rate  für die Gruppe R. Stehen weder Medikamente noch eine Impfung zur Verfügung, so kann man nur die Zahl der Infektionen reduzieren (häufig als „Abflachung der Kurve“ bezeichnet), indem man geeignete Maßnahmen ergreift (z. B. durch weitgehende Kontaktvermeidung, Ausgangsperre). Die Animation zeigt, wie sich eine Senkung der Infektionsrate um 76 % (von für die Gruppe R. Stehen weder Medikamente noch eine Impfung zur Verfügung, so kann man nur die Zahl der Infektionen reduzieren (häufig als „Abflachung der Kurve“ bezeichnet), indem man geeignete Maßnahmen ergreift (z. B. durch weitgehende Kontaktvermeidung, Ausgangsperre). Die Animation zeigt, wie sich eine Senkung der Infektionsrate um 76 % (von  auf auf  ) auswirkt. System von Differentialgleichungen für dieses Modell: ) auswirkt. System von Differentialgleichungen für dieses Modell:
Diese Animation wurde mit GeoGebra erstellt, wobei die numerischen Lösungen des genannten Systems der Differentialgleichungen ermittelt wurden. Der entscheidende Teil der Konstruktion lautet: ##########################################
# das System der Differentialgleichungen #
##########################################
S'(t, S, I, R) = -ß I S
I'(t, S, I, R) = ß S I - γ I
R'(t, S, I, R) = γ I
#######################################################################
# numerische Lösung des Systems der Differentialgleichungen bestimmen #
#######################################################################
NLöseDgl[{S', I', R'}, 0, {s_0, i_0, r_0}, T_{max}]
# liefert:
# NumerischesIntegral1 -> S
# NumerischesIntegral2 -> I
# NumerischesIntegral3 -> RAnmerkung: In der Literatur werden teilweise modifizierte Formen dieser Differentialgleichungen (DGL) benutzt, die aber gleichwertig sind. Leider werden oft dieselben Parameter benutzt. Beispiel für die erste DGL, wobei die Infektionsrate der Klarheit halber  , so ist die DGL identisch mit der oben verwendeten. , so ist die DGL identisch mit der oben verwendeten.  ist konstant (Invariante des Modells) und für die Aufstellung der DGL nicht erforderlich; bei der hier verwendeten Implementierung wurde noch nicht einmal eine Variable für ist konstant (Invariante des Modells) und für die Aufstellung der DGL nicht erforderlich; bei der hier verwendeten Implementierung wurde noch nicht einmal eine Variable für  vorgesehen. vorgesehen.English: Animation of the SIR model with initial values  , ,  , ,  , and rate of recovery , and rate of recovery  . The animation shows the effect of reducing the rate of infection from . The animation shows the effect of reducing the rate of infection from  to to  . If there is no medicine or vaccination available, it is only possible to reduce the infection rate (often referred to as "flattening the curve") by appropriate measures such as social distancing. System of differential equations used for this model: . If there is no medicine or vaccination available, it is only possible to reduce the infection rate (often referred to as "flattening the curve") by appropriate measures such as social distancing. System of differential equations used for this model:
This animation was created with GeoGebra by computing the numerical solutions of the system of differential equations. The core part of the construction is as follows: ####################################
# system of differential equations #
####################################
S'(t, S, I, R) = -ß I S
I'(t, S, I, R) = ß S I - γ I
R'(t, S, I, R) = γ I
###############################################################
# numerical solutions of the system of differential equations #
###############################################################
NSolveODE[{S', I', R'}, 0, {s_0, i_0, r_0}, T_{max}]
# Result:
# numericalIntegral1 -> S
# numericalIntegral2 -> I
# numericalIntegral3 -> RNote: Some authors use modified, but equivalent forms of the ODEs. Unfortunately, the same parameters are sometimes used. Example for the first ODE, where the infection rate is named  , you get the same ODE as above. , you get the same ODE as above.  is a constant value (invariant of the model) and not needed for the ODE; in fact, the implementation didn't even use a variable for is a constant value (invariant of the model) and not needed for the ODE; in fact, the implementation didn't even use a variable for  . . |
| Date | |
| Source | own image |
| Author | Phrontis |
Licensing
[edit]- You are free:
- to share – to copy, distribute and transmit the work
- to remix – to adapt the work
- Under the following conditions:
- attribution – You must give appropriate credit, provide a link to the license, and indicate if changes were made. You may do so in any reasonable manner, but not in any way that suggests the licensor endorses you or your use.
- share alike – If you remix, transform, or build upon the material, you must distribute your contributions under the same or compatible license as the original.
 I have published this image or video as author Phrontis under the licence "CC-BY-SA-3.0" in Wikipedia. This means that free (non-commercial as well as commercial) usage outside of Wikipedia is permitted under the following licence terms:
Please send me a specimen copy or the URL of the website where the image or video is used. Please contact me via Wikipedia (email (account needed) or leave a message) if you
|
 Dieses Foto bzw. Video habe ich als Urheber Phrontis unter der Lizenz „CC-BY-SA-3.0 (de)“ in der Wikipedia veröffentlicht. Dies bedeutet, dass eine kostenlose – sowohl nichtkommerzielle als auch kommerzielle – Nutzung außerhalb der Wikipedia unter folgenden Bedingungen möglich ist:
Ich bitte um Zusendung eines Belegexemplares bzw. der URL, wo das Foto bzw. das Video benutzt wird. Bitte kontaktieren Sie mich über Wikipedia (E-Mail (Benutzerkonto erforderlich) oder hinterlassen Sie eine Nachricht), wenn Sie
|
File history
Click on a date/time to view the file as it appeared at that time.
| Date/Time | Thumbnail | Dimensions | User | Comment | |
|---|---|---|---|---|---|
| current | 09:52, 28 March 2020 |  | 1,048 × 827 (4.62 MB) | Phrontis (talk | contribs) | +loop |
| 12:47, 18 March 2020 |  | 1,048 × 827 (4.62 MB) | Phrontis (talk | contribs) | == {{int:filedesc}} == {{Information |Description= {{de|1=Animation zum SIR-Modell mit den Startwerten <math display="inline">S_0=997, I_0=3, R_0=0</math> sowie anfänglicher Infektionsrate <math display="inline">\beta=0,0005</math> sowie der konstanten Rate <math display="inline">\gamma=0,004</math> für die Gruppe ''R''. Stehen weder Medikamente noch eine Impfung zur Verfügung, so kann man nur die Zahl der Infektionen reduzieren (häufig als „Abflachung der Kurve“ bezeichnet), indem man geeig... |
You cannot overwrite this file.
File usage on Commons
There are no pages that use this file.
File usage on other wikis
The following other wikis use this file:
- Usage on bjn.wikipedia.org
- Usage on de.wikipedia.org
- Usage on en.wikipedia.org
- Usage on en.wikiversity.org
- Usage on fi.wikipedia.org
- Usage on he.wikipedia.org
- Usage on id.wikipedia.org
- Usage on it.wikipedia.org
- Usage on ms.wikipedia.org
- Usage on no.wikipedia.org
- Usage on pl.wikipedia.org
- Usage on uk.wikipedia.org
- Usage on vi.wikipedia.org








